Blog
2017-02-25
Recently, I've noticed a few great examples of misleading uses of numbers in news articles.
On 15 Feb, BBC News published a breaking news article with the headline
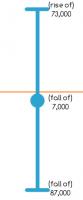
"UK unemployment falls by 7,000 to 1.6m".
This fall of 7,000 sounds big; but when compared to the total of 1.6m, it
is insignificant. The change could more accurately be described as a fall from 1.6m to 1.6m.
But there is a greater problem with this figure. In the
original Office of National Statistics (ONS) report,
the fall of 7,000 was accompanied by a 95% confidence interval of ±80,000.
When calculating figures about large populations (such as unemployment levels), it is impossible to ask every person in the UK whether they
are employed or not. Instead, data is gathered from a sample and this is used to estimate the total number. The 95% confidence interval
gives an idea of the accuracy of this estimation: 95% of the time, the true number will lie of the confidence interval. Therefore, we can
think of the 95% confidence interval as being a range in which the figure lies (although this is not true, it is a helpful way to think
about it).
Compared to the size of its confidence interval (±80,000), the fall of 7,000 is almost indistinguishable from zero. This means that it
cannot be said with any confidence whether the unemployment level rose or fell. This is demonstrated in the following diagram.
![](javascript:showlimage("errorbars1.jpg"))
A fall of 7,000 ± 80,000. The orange line shows no change.
To be fair to the BBC, the headline of the article changed to "UK wage growth outpaces inflation"
once the article was upgraded from breaking news to a complete article, and a mention of the lack of confidence in the change was added.
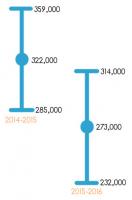
On 23 Feb, I noticed another BBC News with misleading figures: Net migration to UK falls by 49,000.
This 49,000 is the difference between
322,000 (net migration for the year ending 2015) and
273,000 (net migration for the year ending 2016).
However both these figures are estimates: in the original ONS report,
they were placed in 95% confidence intervals of ±37,000 and ±41,000 respectively. As can be seen in the diagram below,
there is a significant portion where these intervals overlap, so it cannot be said with any confidence whether or not net immigration actually fell.
![](javascript:showlimage("errorbars2.jpg"))
Net migration in 2014-15 and 2015-16.
Perhaps the blame for this questionable figure lies with the ONS, as it appeared prominently in their report while the discussion of its
accuracy was fairly well hidden. Although I can't shift all blame from the journalists: they should really be investigating the quality of these
figures, however well advertised their accuracy is.
Both articles criticised here appeared on BBC News. This is not due to the BBC being especially bad with figures, but simply due to the
fact that I spend more time reading news on the BBC than in other places, so noticed these figures there. I quick Google search reveals that the unemployment figure was
also reported, with little to no discussion of accuracy, by
The Guardian,
the Financial Times, and
Sky News.
×2 ×2 ×2 ×2 ×2(Click on one of these icons to react to this blog post)
You might also enjoy...
Comments
Comments in green were written by me. Comments in blue were not written by me.
Add a Comment
2017-01-13
I wrote this post with, and after much discussion with Adam Townsend. It also appeared on the Chalkdust Magazine blog.
Recently, Colin "IceCol" Beveridge blogged about something that's been irking him for a while: those annoying social media posts that tell you to work out a sum, such as \(3-3\times6+2\), and state that only $n$% of people will get it right (where \(n\) is quite small). Or as he calls it "fake maths".
![](javascript:showlimage("fake-maths.jpg"))
A classic example of "fake maths".
This got me thinking about everyone's least favourite primary school acronym: BODMAS (sometimes known as BIDMAS, or PEMDAS if you're American). As I'm sure you've been trying to forget, BODMAS stands for "Brackets, (to the power) Of, Division, Multiplication, Addition, Subtraction" and tells you in which order the operations should be performed.
Now, I agree that we all need to do operations in the same order (just imagine trying to explain your working out to someone who uses BADSOM!) but BODMAS isn't the order mathematicians use. It's simply wrong. Take the sum \(4-3+1\) as an example. Anyone can tell you that the answer is 2. But BODMAS begs to differ: addition comes first, giving 0!
The problem here is that in reality, we treat addition and subtraction as equally important, so sums involving just these two operations are calculated from left-to-right. This caveat is quite a lot more to remember on top of BODMAS, but there's actually no need: Doing all the subtractions before additions will always give you the same answer as going from left-to-right. The same applies to division and multiplication, but luckily these two are in the correct order already in BODMAS (but no luck if you're using PEMDAS).
So instead of BODMAS, we should be using BODMSA. But that's unpronounceable, so instead we suggest that from now on you use MEDUSA. That's right, MEDUSA:
- Mabano (brackets in Swahili)
- Exponentiation
- Division
- Ukubuyabuyelela (multiplication in Zulu)
- Subtraction
- Addition
This is big news. MEDUSA vs BODMAS could be this year's pi vs tau... Although it's not actually the biggest issue when considering sums like \(3-3\times6+2\).
The real problem with \(3-3\times6+2\) is that it is written in a purposefully confusing and ambiguous order. Compare the following sums:
$$3-3\times6+2$$ $$3+2-3\times6$$ $$3+2-(3\times6)$$
In the latter two, it is much harder to make a mistake in the order of operations, because the correct order is much closer to normal left-to-right reading order, helping the reader to avoid common mistakes. Good mathematics is about good communication, not tricking people. This is why questions like this are "fake maths": real mathematicians would never ask them. If we take the time to write clearly, then I bet more than \(n\)% of people will be able get the correct answer.
×1 ×1 ×1 ×1 ×1(Click on one of these icons to react to this blog post)
You might also enjoy...
Comments
Comments in green were written by me. Comments in blue were not written by me.
We use BEDMAS in Canada (Brackets, Exponents, Division, Multiplication, Addition, Subtraction) But we are taught that you do whichever comes first from left to right if they are the addition/ subtraction or multiplication/division. So it could also be BEMDAS, or BEMDSA, or BEDMSA. It just uses the order the that rolls off the tongue more.×1 ×1 ×1 ×1 ×1
Brodaha
×1 ×1 ×1 ×1 ×1 we use BOMAL - Brackets, Overs, Multiplication/Division, Addition/Subtraction, Left to Right. I agree they need to know negative numbers to fully understand and use BODMAS, BIDMAS, BEDMAS, PODMAS, PIDMAS, PEDMAS, BOMAL or MEDUSA×1 ×1 ×1 ×1 ×1
tiny
×1 ×1 ×1 ×1 ×1 If we could just teach young children about positive and negative numbers, then this wouldn't be a problem. Subtraction is just the addition of negative numbers. Division is also the multiplication of fractions. This is why BOMA/PEMA is the optimal method. I think MEDUSA is very creative, though.×1 ×1 ×1 ×1 ×1
Blan
×1 ×1 ×1 ×1 ×1 Add a Comment
2016-12-28
More than ten correct solutions to this year's Advent calendar puzzle competition were submitted on Christmas Day, so the competition is now over.
(Although you can still submit your answers to get me to check them.) Thank-you to everyone who took part in the puzzle, I've had a lot of
fun watching your progress and talking to you on Twitter, Reddit, etc. You can find all the puzzles and answers (from 1 January) here.
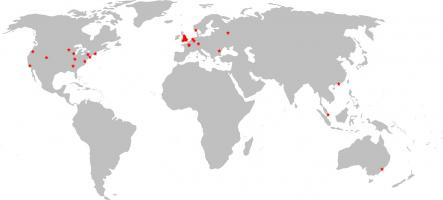
The (very) approximate locations of all the entries I have received so far are shown on this map:
![](javascript:showlimage("advent2016map.jpg"))
This year's winners have been randomly selected from the 29 correct entries on Christmas Day. They are:
| 1 | Jack Jiang |
| 2 | Steve Paget |
| 3 | Joe Gage |
| 4 | Tony Mann |
| 5 | Stephen Cappella |
| 6 | Cheng Wai Koo |
| 7 | Demi Xin |
| 8 | Lyra |
| 9 | David Fox |
| 10 | Bob Dinnage |
Your prizes will be on their way in early January.
Now that the competition has ended, I can give away a secret. Last year, Neal
suggested that it would be fun if a binary picture was hidden in the answers. So this year I hid one. If you write all the answers in binary, with
each answer below the previous and colour in the 1s black, you will see this:
![](javascript:showlimage("advent2016secret.jpg"))
I also had a lot of fun this year making up the names, locations, weapons and motives for the final murder mystery puzzle. In case you missed them these were:
| # | Murder suspect | Motive |
| 1 | Dr. Uno (uno = Spanish 1) | Obeying nameless entity |
| 2 | Mr. Zwei (zwei = German 2) | To worry others |
| 3 | Ms. Trois (trois = French 3) | To help really evil elephant |
| 4 | Mrs. Quattro (quattro = Italian 4) | For old unknown reasons |
| 5 | Prof. Pum (pum = Welsh 5) | For individual violent end |
| 6 | Miss. Zes (zes = Dutch 6) | Stopping idiotic xenophobia |
| 7 | Lord Seacht (seacht = Irish 7) | Suspect espied victim eating newlyweds |
| 8 | Lady Oito (oito = Portuguese 8) | Epic insanity got him today |
| 9 | Rev. Novem (novem = Latin 9) | Nobody in newsroom expected |
| # | Location | Weapon |
| 1 | Throne room | Wrench (1 vowel) |
| 2 | Network room | Rope (2 vowels) |
| 3 | Beneath reeds | Revolver (3 vowels) |
| 4 | Edge of our garden | Lead pipe (4 vowels) |
| 5 | Fives court | Neighbour's sword (5 vowels) |
| 6 | On the sixth floor | Super banana bomb (6 vowels) |
| 7 | Sparse venue | Antique candlestick (7 vowels) |
| 8 | Weightlifting room | A foul tasting poison (8 vowels) |
| 9 | Mathematics mezzanine | Run over with an old Ford Focus (9 vowels) |
Finally, well done to Scott,
Matthew Schulz,
Michael Gustin,
Daniel Branscombe,
Kei Nishimura-Gasparian,
Henry Hung,
Mark Fisher,
Jon Palin,
Thomas Tu,
Félix Breton,
Matt Hutton,
Miguel,
Fred Verheul,
Martine Vijn Nome,
Brennan Dolson,
Louis de Mendonca,
Roni,
Dylan Hendrickson,
Martin Harris,
Virgile Andreani,
Valentin Valciu,
and Adia Batic for submitting the correct answer but being too unlucky to win prizes this year. Thank you all for taking part and I'll see you
next December for the next competition.
(Click on one of these icons to react to this blog post)
You might also enjoy...
Comments
Comments in green were written by me. Comments in blue were not written by me.
I got my prize in the mail today. I really liked the stories from Gustave Verbeek; I thought that was pretty clever. I really appreciate you being willing to send the prizes internationally.
Thanks for setting this all up; I had a lot of fun solving the puzzles every day (and solving half them again when my cookie for the site somehow got deleted). I'll be sure to participate next time too!×1
Thanks for setting this all up; I had a lot of fun solving the puzzles every day (and solving half them again when my cookie for the site somehow got deleted). I'll be sure to participate next time too!
SC
×1 Thanks, Matthew! The puzzles were really fun, and piecing the clues was very interesting too!×1
Jack
×1 Add a Comment
2016-12-23
In many early arcade games, the size of the playable area was limited by the size of the screen. To make this area seem larger, or to
make gameplay more interesting, many games used wraparound; allowing the player to leave one side of the screen and return on another.
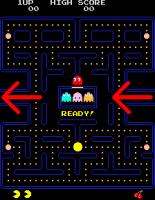
In Pac-Man, for example, the player could leave the left of the screen along the arrow shown and return
on the right, or vice versa.
![](javascript:showlimage("pac-arrows.png"))
Pac-Man's apparent teleportation from one side of the screen to the other may seem like magic, but it is more easily explained by
the shape of Pac-Man's world being a cylinder.
![](javascript:showlimage("pac-cylinder.png"))
Rather than jumping or teleporting from one side to the other, Pac-Man simply travels round the cylinder.
Bubble Bobble was first released in 1986 and features two dragons, Bub and Bob, who are tasked with
rescuing their girlfriends by trapping 100 levels
worth of monsters inside bubbles. In these levels, the dragons and monsters may leave the bottom of the screen to return at the top.
Just like in Pac-Man, Bub and Bob live on the surface of a cylinder, but this time it's horizontal not vertical.
![](javascript:showlimage("bubbob-flat.png"))
![](javascript:showlimage("bubbob-cylinder.png"))
A very large number of arcade games use left-right or top-bottom wrapping and have the same cylindrical shape as Pac-Man or Bubble Bobble.
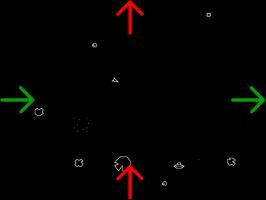
In Asteroids, both left-right and top-bottom wrapping are used.
![](javascript:showlimage("asteroids-arrows.png"))
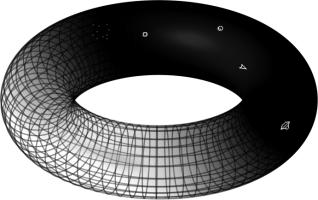
The ships and asteroids in Asteroids live on the surface of a torus, or doughnut: a cylinder around to make its two ends meet up.
![](javascript:showlimage("asteroids-torus.png"))
There is, however, a problem with the torus show here. In Asteroids, the ship will take amount of time to get from the left of the screen
to the right however high or low on the screen it is. But the ship can get around the inside of the torus shown faster than it can
around the outside, as the inside is shorter. This is because the screen of play is completely flat, while the inside and outside halves of
the torus are curved.
It is impossible to make a flat torus in three-dimensional space, but it is possible to make one in
four-dimensional space.
Therefore, while Asteroids seems to be a simple two-dimensional game, it is actually taking place on a four-dimensional surface.
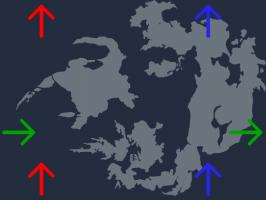
Wrapping doesn't only appear in arcade games. Many games in the excellent Final Fantasy series use wrapping on the world maps, as shown here
on the Final Fantasy VIII map.
![](javascript:showlimage("ff8-arrows.png"))
Just like in Asteroids, this wrapping means that Squall & co. carry out their adventure on the surface of a four-dimensional flat torus.
The game designers, however, seem to not have realised this, as shown in this screenshot including a spherical (!) map.
![](javascript:showlimage("ff8-sphere.png"))
Due to the curvature of a sphere, lines that start off parallel eventually meet. This makes it impossible to map
nicely between a flat surface to a sphere (this is why so many different map projections exist), and heavily complicates the task of making
a game with a truly spherical map. So I'll let the Final Fantasy VIII game designers off. Especially since the rest of the game is such
incredible fun.
It is sad, however, that there are no games (at leat that I know of) that make use of the great variety of different wrapping rules available. By only
slightly adjusting the wrapping rules used in the games in this post, it is possible to make games on a variety of other surfaces,
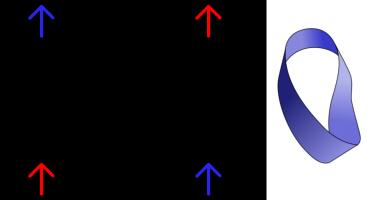
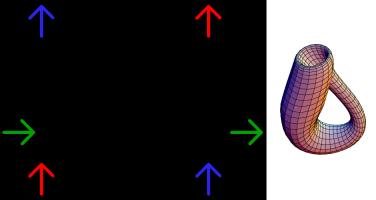
such a Klein bottles or Möbius strips as shown below.
![](javascript:showlimage("mobius-video-game.png"))
![](javascript:showlimage("klein-video-game.png"))
If you know of any games make use of these surfaces, let me know in the comments below!
×1 ×1 ×1 ×1 ×1(Click on one of these icons to react to this blog post)
You might also enjoy...
Comments
Comments in green were written by me. Comments in blue were not written by me.
HyperRogue also has special modes which experiment with other geometries (spherical, and elliptic which is non-orientable). Hydra Slayer has Mobius strip and Klein bottle levels.×1 ×1 ×1
Zeno Rogue
×1 ×1 ×1 HyperRogue is an example of a game that takes place on the hyperbolic plane. Rather than looping, the map is infinite.
See: http://zenorogue.blogspot.com.au/2012/...
See: http://zenorogue.blogspot.com.au/2012/...
maetl
Hyperrogue may be the only game in existence with a hyperbolic surface topology: http://www.roguetemple.com/z/hyper/
zaratustra
F-Zero GX had a track called Mobius Ring, that was... well, a Möbius ring.
F-Zero X had a more trivial track that was just the outward side of a regular ring, but it was rather weird too, because it meant that this was a looping track that had no turns.
F-Zero X had a more trivial track that was just the outward side of a regular ring, but it was rather weird too, because it meant that this was a looping track that had no turns.
Olaf
Add a Comment
2016-12-20
Last week, I posted about the Christmas card I designed on the Chalkdust blog.
![](javascript:showlimage("card2016.jpg"))
The card looks boring at first glance, but contains 12 puzzles. Converting the answers to base 3, writing them in the boxes on the front, then colouring the 1s green and 2s red will reveal a Christmassy picture.
If you want to try the card yourself, you can download this pdf. Alternatively, you can find the puzzles below and type the answers in the boxes. The answers will be automatically converted to base 3 and coloured...
| # | Answer (base 10) | Answer (base 3) | ||||||||
| 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 3 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 4 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 5 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 6 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 7 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 8 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 9 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 10 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 11 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 12 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
- The square number larger than 1 whose square root is equal to the sum of its digits.
- The smallest square number whose factors add up to a different square number.
- The largest number that cannot be written in the form \(23n+17m\), where \(n\) and \(m\) are positive integers (or 0).
- Write down a three-digit number whose digits are decreasing. Write down the reverse of this number and find the difference. Add this difference to its reverse. What is the result?
- The number of numbers between 0 and 10,000,000 that do not contain the digits 0, 1, 2, 3, 4, 5 or 6.
- The lowest common multiple of 57 and 249.
- The sum of all the odd numbers between 0 and 66.
- One less than four times the 40th triangle number.
- The number of factors of the number \(2^{756}\)×\(3^{12}\).
- In a book with 13,204 pages, what do the page numbers of the middle two pages add up to?
- The number of off-diagonal elements in a 27×27 matrix.
- The largest number, \(k\), such that \(27k/(27+k)\) is an integer.
(Click on one of these icons to react to this blog post)
You might also enjoy...
Comments
Comments in green were written by me. Comments in blue were not written by me.
@Matthew: Thank you for the prompt response! It makes sense now and perhaps I should have read a little closer!
Dan Whitman
@Dan Whitman: Find the difference between the original number and the reverse of the original. Call this difference \(a\). Next add \(a\) to the reverse of \(a\)... ×1
Matthew
×1 In number 4 what are we to take the difference between? Do you mean the difference between the original number and its reverse? If so when you add the difference back to the reverse you simply get the original number, which is ambiguous. I am not sure what you are asking us to do here.
Dan Whitman
Add a Comment
My favorite species of ignoring the measurement error is the metric conversion taken to way too many decimal places. The hike was 50 miles (80.467 kilometers) long.