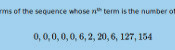Blog
2015-08-29
A few weeks ago, I made OEISbot, a Reddit bot which posts information whenever an OEIS sequence is mentioned.
This post explains how OEISbot works. The full code can be found on GitHub.
Getting started
OEISbot is made in Python using PRAW (Python Reddit Api Wrapper). PRAW can be installed with:
bash
pip install prawBefore making a bot, you will need to make a Reddit account for your bot, create a Reddit app and obtain API keys. This python script can be used to obtain the necessary keys.
Once you have your API keys saved in your praw.ini file, you are ready to make a bot.
Writing the bot
First, the necessary imports are made, and test mode is activated if the script is run with test as an argument. We also define an exception that will be used later to kill the script once it makes a comment.
python
import prawimport re
import urllib
import json
from praw.objects import MoreComments
import sys
test = False
if len(sys.argv) > 1 and sys.argv[1] == "test":
test = True
print("TEST MODE")
class FoundOne(BaseException):
pass
To prevent OEISbot from posting multiple links to the same sequence in a thread, lists of sequences linked to in each thread can be loaded and saved using the following functions.
python
def save_list(seen, _id):print(seen)
with open("/home/pi/OEISbot/seen/"+_id, "w") as f:
return json.dump(seen, f)
def open_list(_id):
try:
with open("/home/pi/OEISbot/seen/" + _id) as f:
return json.load(f)
except:
return []
The following function will search a post for a mention of an OEIS sequence number.
python
def look_for_A(id_, text, url, comment):seen = open_list(id_)
re_s = re.findall("A([0-9]{6})", text)
re_s += re.findall("oeis\.org/A([0-9]{6})", url)
if test:
print(re_s)
post_me = []
for seq_n in re_s:
if seq_n not in seen:
post_me.append(markup(seq_n))
seen.append(seq_n)
if len(post_me) > 0:
post_me.append(me())
comment(joiner().join(post_me))
save_list(seen, id_)
raise FoundOne
The following function will search a post for a comma-separated list of numbers, then search for it on the OEIS. If there are 14 sequences or less found, it will reply. If it finds a list with no matches on the OEIS, it will message /u/PeteOK, as he likes hearing about possibly new sequences.
python
def look_for_ls(id_, text, comment, link, message):seen = open_list(id_)
if test:
print(text)
re_s = re.findall("([0-9]+\, *(?:[0-9]+\, *)+[0-9]+)", text)
if len(re_s) > 0:
for terms in ["".join(i.split(" ")) for i in re_s]:
if test:
print(terms)
if terms not in seen:
seen.append(terms)
first10, total = load_search(terms)
if test:
print(first10)
if len(first10)>0 and total <= 14:
if total == 1:
intro = "Your sequence (" + terms \
+ ") looks like the following OEIS sequence."
else:
intro = "Your sequence (" + terms + \
+ ") may be one of the following OEIS sequences."
if total > 4:
intro += " Or, it may be one of the " + str(total-4) \
+ " other sequences listed [here]" \
"(http://oeis.org/search?q=" + terms + ")."
post_me = [intro]
if test:
print(first10)
for seq_n in first10[:4]:
post_me.append(markup(seq_n))
seen.append(seq_n)
post_me.append(me())
comment(joiner().join(post_me))
save_list(seen, id_)
raise FoundOne
elif len(first10) == 0:
post_me = ["I couldn't find your sequence (" + terms \
+ ") in the [OEIS](http://oeis.org). "
"You should add it!"]
message("PeteOK",
"Sequence not in OEIS",
"Hi Peter, I've just found a new sequence (" \
+ terms + ") in [this thread](link). " \
"Please shout at /u/mscroggs to turn the " \
"feature off if its spamming you!")
post_me.append(me())
comment(joiner().join(post_me))
save_list(seen, id_)
raise FoundOne
def load_search(terms):
src = urllib.urlopen("http://oeis.org/search?fmt=data&q="+terms).read()
ls = re.findall("href=(?:'|\")/A([0-9]{6})(?:'|\")", src)
try:
tot = int(re.findall("of ([0-9]+) results found", src)[0])
except:
tot = 0
return ls, tot
The markup function loads the necessary information from OEIS and formats it. Each comment will end with the output of the me function. The ouput of joiner will be used between sequences which are mentioned.
python
def markup(seq_n):pattern = re.compile("%N (.*?)<", re.DOTALL|re.M)
desc = urllib.urlopen("http://oeis.org/A" + seq_n + "/internal").read()
desc = pattern.findall(desc)[0].strip("\n")
pattern = re.compile("%S (.*?)<", re.DOTALL|re.M)
seq = urllib.urlopen("http://oeis.org/A" + seq_n + "/internal").read()
seq = pattern.findall(seq)[0].strip("\n")
new_com = "[A" + seq_n + "](http://oeis.org/A" + seq_n + "/): "
new_com += desc + "\n\n"
new_com += seq + "..."
return new_com
def me():
return "I am OEISbot. I was programmed by /u/mscroggs. " \
"[How I work](http://mscroggs.co.uk/blog/20). " \
"You can test me and suggest new features at /r/TestingOEISbot/."
def joiner():
return "\n\n- - - -\n\n"
Next, OEISbot logs into Reddit.
python
r = praw.Reddit("OEIS link and description poster by /u/mscroggs.")access_i = r.refresh_access_information(refresh_token=r.refresh_token)
r.set_access_credentials(**access_i)
auth = r.get_me()
The subs which OEISbot will search through are listed. I have used all the math(s) subs which I know about, as these will be the ones mentioning sequences.
python
subs = ["TestingOEISbot","math","mathpuzzles","casualmath","theydidthemath","learnmath","mathbooks","cheatatmathhomework","matheducation",
"puremathematics","mathpics","mathriddles","askmath",
"recreationalmath","OEIS","mathclubs","maths"]
if test:
subs = ["TestingOEISbot"]
For each sub OEISbot is monitoring, the hottest 10 posts are searched through for mentions of sequences. If a mention is found, a reply is generated and posted, then the FoundOne exception will be raised to end the code.
python
try:for sub in subs:
print(sub)
subreddit = r.get_subreddit(sub)
for submission in subreddit.get_hot(limit = 10):
if test:
print(submission.title)
look_for_A(submission.id,
submission.title + "|" + submission.selftext,
submission.url,
submission.add_comment)
look_for_ls(submission.id,
submission.title + "|" + submission.selftext,
submission.add_comment,
submission.url,
r.send_message)
flat_comments = praw.helpers.flatten_tree(submission.comments)
for comment in flat_comments:
if ( not isinstance(comment, MoreComments)
and comment.author is not None
and comment.author.name != "OEISbot" ):
look_for_A(submission.id,
re.sub("\[[^\]]*\]\([^\)*]\)","",comment.body),
comment.body,
comment.reply)
look_for_ls(submission.id,
re.sub("\[[^\]]*\]\([^\)*]\)","",comment.body),
comment.reply,
submission.url,
r.send_message)
except FoundOne:
pass
Running the code
I put this script on a Raspberry Pi which runs it every 10 minutes (to prevent OEISbot from getting refusals for posting too often). This is achieved with a cron job.
bash
*/10 * * * * python /path/to/bot.pyMaking your own bot
The full OEISbot code is available on GitHub. Feel free to use it as a starting point to make your own bot! If your bot is successful, let me know about it in the comments below or on Twitter.
Edit: Updated to describe the latest version of OEISbot.
(Click on one of these icons to react to this blog post)
You might also enjoy...
Comments
Comments in green were written by me. Comments in blue were not written by me.
Add a Comment
2015-03-15
A few months ago, I set
@mathslogicbot (and @logicbot@mathstodon.xyz and @logicbot.bsky.social) going on the
long task of tweeting all the tautologies (containing 140 characters or less)
in propositional calculus with the symbols \(\neg\) (not), \(\rightarrow\)
(implies), \(\leftrightarrow\) (if and only if), \(\wedge\) (and) and \(\vee\)
(or). My first post on logic bot contains a full
explanation of propositional calculus, formulae and tautologies.
An alternative method
Since writing the original post, I have written an alternative script to
generate all the tautologies.
In this new method, I run through all possible strings of length 1 made
with character in the logical language, then strings of length 2, 3 and so on.
The script then checks if they are valid formulae and, if so, if they are
tautologies.
In the new script, only formulae where the first appearances of variables
are in alphabetical order are considered. This means that duplicate tautologies
are removed. For example, \((b\rightarrow(b\wedge a))\) will now be counted as
it is the same as \((a\rightarrow(a\wedge b))\).
You can view or download this alternative code on
github.
All the terms of the sequence that I have calculated so far can be viewed
here and the tautologies for these terms are
here.
Sequence
One advantage of this method is that it generates the tautologies sorted by
the number of symbols they contain, meaning we can generate the sequence whose
\(n\)th term is the number of tautologies of length \(n\).
The first ten terms of this sequence are
$$0, 0, 0, 0, 2, 2, 12, 6, 57, 88$$
as there are no tautologies of length less than 5; and, for example two
tautologies of length 6 (\((\neg a\vee a)\) and \((a\vee \neg a)\)).
This sequence is listed as
A256120 on OEIS.
Properties
There are a few properties of this sequence that can easily be shown.
Throughout this section I will use \(a_n\) to represent the \(n\)th
term of the sequence.
Firstly, \(a_{n+2}\geq a_n\). This can be explained as follows: let \(A\)
be a tautology of length \(n\). \(\neg\neg A\) will be of length \(n+2\) and
is logically equivalent to \(A\).
Another property is \(a_{n+4}\geq 2a_n\): given a tautology \(A\) of length
\(n\), both \((a\vee A)\) and \((A\vee a)\) will be tautologies of length
\(n+4\). Similar properties could be shown for \(\rightarrow\),
\(\leftrightarrow\) and \(\wedge\).
Given properties like this, one might predict that the sequence will be
increasing (\(a_{n+1}\geq a_n\)). However this is not true as \(a_7\) is 12
and \(a_8\) is only 6. It would be interesting to know at how many points in
the sequence there is a term that is less than the previous one. Given the
properties above it is reasonable to conjecture that this is the only one.
Edit: The sequence has been published on OEIS!
Edit: Added Mastodon and Bluesky links
(Click on one of these icons to react to this blog post)
You might also enjoy...
Comments
Comments in green were written by me. Comments in blue were not written by me.
Great project! Would be interesting to have a version of this for the sheffer stroke.
om
Add a Comment