Puzzles
Half an equilateral triangle
What is the shortest straight line which bisects an equilateral triangle?
Show answer & extension
Hide answer & extension
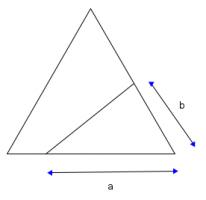
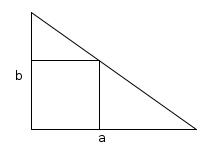
Consider a line which bisects an equilateral triangle with sides of length 1. Let the sides of the smaller triangle created be \(a\) and \(b\) as shown below:
The area of the equilateral triangle is:
$$\frac{1}{2}\times 1\times 1\times \sin(60)\\=\frac{\sqrt{3}}{4}$$
The area of the smaller triangle is:
$$\frac{1}{2}ab \sin(60)\\=\frac{ab\sqrt{3}}{4}$$
This is half the area of the equilateral triangle, so:
$$ab=\frac{1}{2}$$
The length (\(L\)) of the line bisecting the equilateral triangle is:
$$L=a^2+b^2-2ab\cos(60)\\
=a^2+\left(\frac{1}{2a}\right)^2-\frac{2a}{2a}\times \frac{1}{2}\\
=a^2+\frac{1}{4a^2}-\frac{1}{2}$$
To find the minimum, we set the derivative to 0:
$$0=\frac{dL}{da}=2a-\frac{1}{2a^3}\\
4a^4-1=0\\
a^4=\frac{1}{4}\\
a=\frac{1}{\sqrt{2}}$$
For this value of \(a\), \(b\) and \(L\) also equal to \(\frac{1}{\sqrt{2}}\).
Extension
What if the line does not need to be straight?
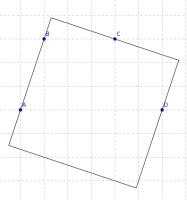
Four points on a shape
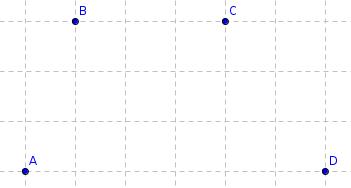
Fiona wants to draw a 2-dimensional shape whose perimeter passes through the points A, B, C and D
Which of the following shapes can she draw?
(i) A circle
(ii) An equilateral triangle
(iii) A square
Show answer & extension
Hide answer & extension
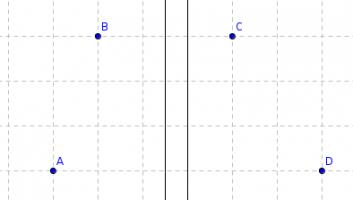
(i) If a circle could be drawn, then its centre would be equidistant from each pair of points. But the locus of points equidistant from B and C is parallel to the locus of points equidistant from A and D.
Therefore it is impossible to place the centre of the circle, so no circle can be drawn.
(ii)
(iii)
Extension
Let A, B, C and D be any four points. When is it possible to draw
(i) A circle
(ii) An equilateral triangle
(iii) A square
through the four points?
Pizza
Twelve friends want to share a pizza. One of the friends is very fussy and will not eat the centre of the pizza.
Is it possible to split a (circular) pizza into twelve identical pieces such that there is at least one piece which does not touch the centre?
Square in a triangle
A right-angled triangle has short sides of length \(a\) and \(b\). A square is drawn in the triangle so that two sides lie on the sides of the triangle and a corner lies on the hypotenuse.
What is the length of a side of the square?
Show answer & extension
Hide answer & extension
Call the length of a side of the square \(x\). CAB and CEF are similar triangles, therefore:
$$\frac{EF}{AB}=\frac{EC}{AC}$$
$$\frac{x}{a}=\frac{b-x}{b}$$
$$bx=ab-ax$$
$$x=\frac{ab}{a+b}$$
Extension
Consider a tetrahedron with vertices at \((0,0,0)\), \((a,0,0)\), \((0,b,0)\) and \((0,0,c)\). A cube is placed in the tetrahedron so that three of its faces are in the xy, xz and yz planes and one of its vertices lies on the other face.
What is the length of a side of the cube?
Three squares
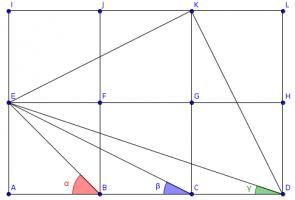
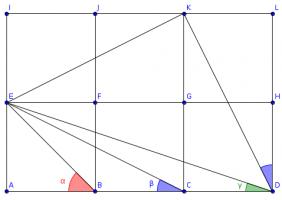
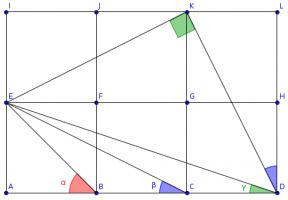
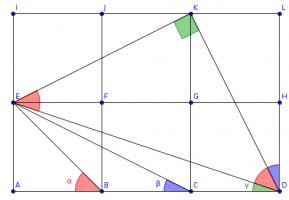
The diagram shows three squares with diagonals drawn on and three angles labelled.
What is the value of \(\alpha+\beta+\gamma\)?
Show answer & extension
Hide answer & extension
Draw three more squares and add these lines (I have coloured the angles to make equal angles clearer):
Triangles \(ACE\), \(LDK\) and \(IKE\) are congruent, so angle \(KDL\) is equal to \(\beta\).
The congruence of these triangles tells us that angles \(DKL\) and \(EKI\) add up to a right angle, so angle \(EKD\) is also a right angle.
The congruence of the triangles also tells us that \(KD\) and \(KE\) are the same length and so angle \(EDK\) is the angle in an isosceles right-angled triangle. \(\alpha\) is also the angle in an isosceles right-angled triangle, so these two angles are equal.
Therefore \(\alpha+\beta+\gamma=90^\circ\).
Extension
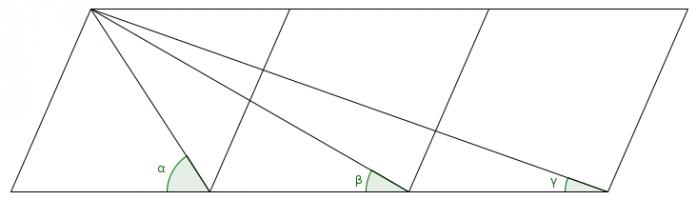
The diagram shows three rhombuses with diagonals drawn on and three angles labelled.
What is the value of \(\alpha+\beta+\gamma\)?
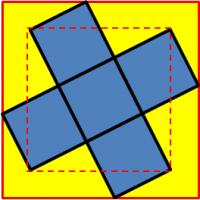
Square cross
A figure in the shape of a cross is made from five 1 x 1 squares, as shown. The cross is inscribed in a large square whose sides are parallel to the dashed square, formed by four vertices of the cross.
What is the area of the large outer square?
Show answer
Hide answer
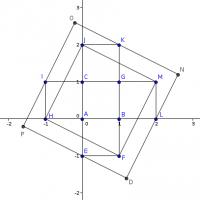
The smaller diagonal square is made up of a 1×1 square and four 1×2 right-angled triangles. Therefore its area is 5.
The line FM, and therefore the line DN, has gradient 2. The line JM, and therefore the line ON, has gradient -½. ON passes through (1,2) and DN passes through (2,0). Therefore, DN has equation \(y=2x-4\) and ON has equation \(y=\frac{5}{2}-\frac{1}{2}x\). These lines intersect at \((\frac{13}{5},\frac{6}{5})\), these are the co-ordinates of N. By the same method, the co-ordinates of P are \((-\frac{8}{5},-\frac{1}{5})\).
By Pythagoras' Theorem, The diagonal of the larger square is \(\frac{7\sqrt{10}}{5}\) and so the area of the larger square is 9.8.
Overlapping triangles
Four congruent triangles are drawn in a square.
The total area which the triangles overlap (red) is equal to the area
they don't cover (blue). What proportion of the area of the large square
does each (purple) triangle take up?

Show answer & extension
Hide answer & extension
Let \(S\) be the area of the large square, \(T\) be the area
of one of the large triangles, \(U\) be one of the red overlaps
and V be the uncovered blue square. We can write
$$S=4T-4U+V$$
as the area of the square is the total of the four triangles,
take away the overlaps as they have been double counted, add
the blue square as it has been missed.
We know that 4U=V, so
$$S=4T-V+V$$
$$S=4T.$$
Therefore one of the triangles covers one quarter of the
square.
Extension
Five congruent triangles are drawn in a regular pentagon. The
total area which the triangles overlap (red) is equal to the area they
don't cover (blue). What proportion of the area of the large pentagon
does each triangle take up?
\(n\) congruent triangles are drawn in a regular \(n\) sided polygon.
The
total
area which the triangles overlap is equal to the area they don't cover.
What proportion of the area of the large \(n\) sided polygon does each
triangle take up?
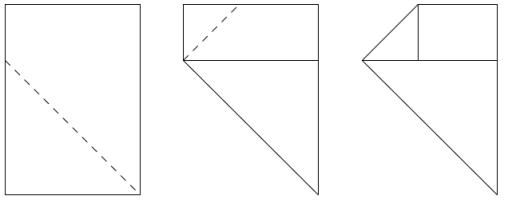
Folding A4 paper
A Piece of
A4 paper is folded as shown:
What shape is made?
Show answer & extension
Hide answer & extension
The sides of A4 paper are in the ratio \(1:\sqrt{2}\). Let the width of the paper be 1 unit. This means that the height of the paper is \(\sqrt{2}\) units.
Therefore on the diagram, \(AE=1, BE=1, AC=\sqrt{2}\). By Pythagoras' Theorem, \(AB=\sqrt{2}\), so \(AB=AC\).
\(BF=DF=\sqrt{2}-1\) so by Pythagoras' Theorem again, \(BD=2-\sqrt{2}\). \(CD=1-(\sqrt{2}-1)=2-\sqrt{2}\). Hence, \(CD=BD\) and so the shape is a kite.
Extension
Prove that for a starting rectangle with the sides in any ratio, the resulting shape is a
cyclic quadrilateral.