Puzzles
Two tangents
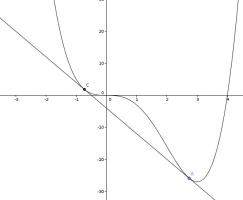
Find a line which is tangent to the curve \(y=x^4-4x^3\) at 2 points.
Show answer
Hide answer
At \(x=a\), \(y=a^4-4a^2\) and \(\frac{dy}{dx}=4a^3-12a^2\). Therefore the equation of the tangent at \(x=a\) will be \(y=(4a^3-12a^2)x+8a^3-3a^4\).
Taking this away from \(y=x^4-4x^3\) gives \(y=x^4-4x^3-(4a^3-12a^2)x-8a^3+3a^4\). We can now look at where this curve is tangent to \(y=0\) and look for a value of \(a\) that makes it tangent at two points.
If this curve is tangent to the \(x\)-axis at \(x=b\), then it will have a repeated root at \(x=b\). We know it is tangent at \(x=a\), so dividing \(x^4-4x^3-(4a^3-12a^2)x-8a^3+3a^4\) by \((x-a)\) twice gives \(x^2+(2a-4)x+3a^2-8a\). We want this to have a repeated root, hence the discriminant, \((2a-4)^2-4(3a^2-8a)\), must be 0.
Solving this gives \(a=1\pm\sqrt3\).
Therefore the equation of the line is \(y=-8x-4\).
Double derivative
What is
$$\frac{d}{dy}\left(\frac{dy}{dx}\right)$$
when:
(i) \(y=x\)
(ii) \(y=x^2\)
(iii) \(y=x^3\)
(iv) \(y=x^n\)
(v) \(y=e^x\)
(vi) \(y=\sin(x)\)?
Show answer & extension
Hide answer & extension
(ii) Differentiating \(y=x^2\) with respect to \(x\) \(\frac{dy}{dx}=2x\). Let \(g=\frac{dy}{dx}\). By the chain rule:
$$\frac{dg}{dy}=\frac{dg}{dx}\frac{dx}{dy}$$
$$=2\frac{1}{2x}$$
$$=\frac{1}{x}$$
So \(\frac{d}{dy}\left(\frac{dy}{dx}\right)=\frac{1}{x}\)
(iii) By the same method, \(\frac{d}{dy}\left(\frac{dy}{dx}\right)=\frac{2}{x}\)
(iv) \(\frac{d}{dy}\left(\frac{dy}{dx}\right)=\frac{n-1}{x}\)
(v) \(\frac{d}{dy}\left(\frac{dy}{dx}\right)=1\)
(vi) \(\frac{d}{dy}\left(\frac{dy}{dx}\right)=-\tan(x)\)
Extension
What is
$$\frac{d}{dy}\left(\frac{dy}{dx}\right)$$
when \(y=f(x)\)?
Differentiate this
$$f(x)=e^{x^{ \frac{\ln{\left(\ln{x}\right)}}{ \ln{x}}} }$$
Find \(f'(x)\).
Show answer
Hide answer
$$f(x)=e^{x^{ \frac{\ln{\left(\ln{x}\right)}}{ \ln{x}}} }$$
$$=e^{e^{ \frac{\ln{\left(\ln{x}\right)}}{ \ln{x}}\ln{x}} }$$
$$=e^{e^{ \ln{\left(\ln{x}\right)}} }$$
$$=e^{\ln{x} }$$
$$=x$$
Therefore:
$$f'(x)=1$$
x to the power of x again
Let \(y=x^{x^{x^{x^{...}}}}\) [\(x\) to the power of (\(x\) to the power of (\(x\) to the power of (\(x\) to the power of ...))) with an infinite number of \(x\)s]. What is \(\frac{dy}{dx}\)?
Show answer & extension
Hide answer & extension
\(y=x^{x^{x^{x^{...}}}}\) so \(y=x^y=e^{y\ln{x}}\).
By the chain and product rules, \(\frac{dy}{dx}=e^{y\ln{x}}(\frac{dy}{dx}\ln{x}+\frac{y}{x})\).
Rearranging, we get \(\frac{dy}{dx}=\frac{ye^{y\ln{x}}}{x(1-e^{y\ln{x}}\ln{x})}\).
This simplifies to \(\frac{dy}{dx}=\frac{x^{x^{x^{x^{...}}}}x^{x^{x^{x^{...}}}}}{x(1-x^{x^{x^{x^{...}}}}\ln{x})}\).
Extension
What would the graph of \(y=x^{x^{x^{x^{...}}}}\) look like?