Puzzles
13 December
Today's number is given in this crossnumber. No number in the completed grid starts with 0.
|
|
| Across |
| 1 | Today's number. | (3) |
| 4 | A multiple of 5 times the sum of the digits of 2D. | (3) |
| 5 | Greater than two times 3D. | (3) |
|
| Down |
| 1 | The sum of 5A and 3D. | (3) |
| 2 | Nine times the sum of the digits of 4A. | (3) |
| 3 | Greater than 2D. | (3) |
|
Show answer
Hide answer
2D is a multiple of 9, and so its digits add up to a multiple of 9. This means that 4A is a multiple of 9, and its digits add up to a multiple of 9 too.
The digits of 4A can add up to at most 27 (if it's 999) and so must add add up to 9, 18, or 27. These would make 2D equal to 81, 162, or 243 respectively. 2D cannot be 81 (as it's a three-digit number);
it also can't be 243, as this would mean 4A was 999, leading to a clash in the centre square. Therefore the sum of the digits of 4A is 18 and 2D is 162:
The sum of the digits of 4A is 18, so 4A is 369, 468, 567, 666, 765, 864, or 963. Of these, only 765 is a multiple of 5, and so 4A must be 765:
3D is greater than 2D, so the first digit of 3D is at least 2. If the first digit of 3D was 3 or higher, then 5A would be greater than 700, meaning 1D would be greater than 1000 and not a three-digit number.
Therefore:
The last digits of 3D and 5A are the same, and so the last digit of 1D (and the first digit of 5A) must be even. 5A is greater than 500, and so it's first digit must be 6 or 8;
it cannot be 8 as this would make 1D greater than 100. And so:
This only leaves one option for the last two digits to make 1D's clue correct:
Today's number is 812.
12 December
What is the smallest value of \(n\) such that
$$\frac{500!\times499!\times498!\times\dots\times1!}{n!}$$
is a square number?
Show answer
Hide answer
Consider the first two terms in the product:
$$
500!\times499!
=500\times499!\times499!$$
$$= 500\times(499!)^2.$$
Doing similar steps with each pair of terms in the product, we see that:
$$
500!\times499!\times498!\times\dots\times1!
=
500\times498\times\dots\times2\times(499!\times497!\times\dots\times1!)^2
$$
$$
=
(2\times250)\times(2\times249)\times\dots\times(2\times1)\times(499!\times497!\times\dots\times1!)^2
$$
$$
=
2^{250}\times250!\times(499!\times497!\times\dots\times1!)^2
$$
If we divide this by \(250!\), we are left with a square number, and so \(n\) is 250
11 December
Put the digits 1 to 9 (using each digit exactly once) in the boxes so that the sums are correct. The sums should be read left to right and top to bottom ignoring the usual order of operations. For example, 4+3×2 is 14, not 10.
Today's number is the product of the numbers in the red boxes.
| + | | + | | = 15 |
| + | | + | | ÷ | |
| + | | – | | = 10 |
| + | | – | | × | |
| ÷ | | × | | = 3 |
=
16 | | =
1 | | =
30 | |
Show answer
Hide answer
| 3 | + | 7 | + | 5 | = 15 |
| + | | + | | ÷ | |
| 9 | + | 2 | – | 1 | = 10 |
| + | | – | | × | |
| 4 | ÷ | 8 | × | 6 | = 3 |
=
16 | | =
1 | | =
30 | |
The product of the numbers in the red boxes is 120.
10 December
How many integers are there between 100 and 1000 whose digits add up to an even number?
Show answer
Hide answer
Between 100 and 109 (inclusive), there are 5 integers whose digits add up to an even number, and 5 whose digits add up to an odd number.
Between 110 and 119 (inclusive), there are 5 integers whose digits add up to an even number, and 5 whose digits add up to an odd number...
In general, between \(10n\) and \(10n+9\) (inclusive), there are 5 integers whose digits add up to an even number, and 5 whose digits add up to an odd number.
The integers from 100 to 999 (inclusive) can be split into 45 sets of integers from \(10n\) to \(10n+9\) (and the digits of 1000 don't add to an even number), so there are
450 integers between 100 and 1000 whose digits add up to an even number.
9 December
The diagram below shows a rectangle. Two of its sides have been coloured blue. A red line has been drawn from two of its vertices to the midpoint of a side.
The total length of the blue lines is 50cm. The total length of the red lines is also 50cm. What is the area of the rectangle (in cm2)?
Show answer
Hide answer
Let \(a\) be the height of the rectangle. As the total of the blue lines is 50, the width of the rectangle is \(50-a\).
As the total of the red lines is 50, each red line segment is 25.
Using Pythagoras's theorem in one of the right-angled triangles, we see that:
$$a^2 + \left(\frac{50-a}{2}\right)^2 = 25^2$$
$$4a^2 + (50-a)^2 = 50^2$$
$$4a^2 + 50^2 - 100a + a^2 = 50^2$$
$$a(5a - 100) = 0$$
\(a\) is not zero, and so \(a=20\). This means that the area of the rectangle is 20×30=600.
8 December
Noel writes the numbers 1 to 17 in a row. Underneath, he writes the same list without the first and last numbers, then continues this until he writes a row containing just one number:
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 |
| | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | |
| | | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | | |
| etc. |
What is the sum of all the numbers that Noel has written?
7 December
There are 8 sets (including the empty set) that contain numbers from 1 to 4 that don't include any consecutive integers:
\(\{\}\), \(\{1\}\), \(\{2\}\), \(\{3\}\), \(\{4\}\), \(\{1,3\}\), \(\{1,4\}\), \(\{2, 4\}\)
How many sets (including the empty set) are there that contain numbers from 1 to 14 that don't include any consecutive integers?
6 December
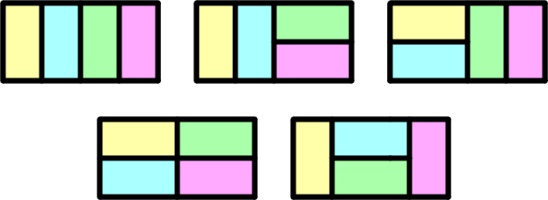
There are 5 ways to tile a 4×2 rectangle with 2×1 pieces:
How many ways are there to tile a 12×2 rectangle with 2×1 pieces?
Show answer
Hide answer
Let \(a_n\) be the number of ways to tile a \(n\times2\) rectangle.
It is easy to check that \(a_1=1\) (ie there is 1 way to tile a 1×2 rectangle) and \(a_2=2\) (ie there are 2 ways to tile a 2×2 rectangle).
For an \(n\times2\) rectangle, from the left the tiling either starts with a vertical tile, or a pair of horizontal tiles.
If it starts with a vertical tile, then there are \(a_{n-1}\) ways to tile the remaining \((n-1)\times2\) rectangle.
If it starts with a pair of horizontal tile2, then there are \(a_{n-2}\) ways to tile the remaining \((n-2)\times2\) rectangle.
Therefore, \(a_n=a_{n-1}+a_{n-2}\).
(And so the number of ways to tile a \(n\times2\) rectangle is the \((n+1)\)th Fibonacci number.)
Therefore, the number of ways to tile a 12×2 rectangle is 233.