Puzzles
Showing old puzzles. Show newest puzzles.
8 December
The residents of Octingham have 8 fingers. Instead of counting in base ten, they count in base eight: the digits of their numbers represent ones, eights, sixty-fours, two-hundred-and-fifty-sixes, etc
instead of ones, tens, hundreds, thousands, etc.
For example, a residents of Octingham would say 12, 22 and 52 instead of our usual numbers 10, 18 and 42.
Today's number is what a resident of Octingham would call 11 squared (where the 11 is also written using the Octingham number system).
Show answer
Hide answer
The Octingham resident's 11 is equal to our number 9. 9 squared is 81. 81 in base eight is 121.
Interestingly, this is the same answer as "just" doing 11 squred in base ten.
7 December
There are 15 dominos that can be made using the numbers 0 to 4 (inclusive):
|
|
|
|
|
|
|
|
|
||||||||||||||||||
|
|
|
|
|
|
The sum of all the numbers on all these dominos is 60.
Today's number is the sum of all the numbers on all the dominos that can be made using the numbers 5 to 10 (inclusive).
Show answer
Hide answer
Each number will appear 7 times: one time paired with the numbers six numbers 5 to 10, plus an extra appearance on the tile containing the same number twice.
The total of all the numbers is therefore 7×(5+6+...+10)=7×45=315.
6 December
There are 12 ways of placing 2 tokens on a 2×4 grid so that no two tokens are next to each other horizonally, vertically or diagonally:
|
|
|
|
|
|
||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
Today's number is the number of ways of placing 5 tokens on a 2×10 grid so that no two tokens are next to each other horizonally, vertically or diagonally.
Show answer
Hide answer
First, consider placing 5 tiles in a 1×9 grid. There is only one way to do this:
| O | O | O | O | O |
To get the number of ways of placing 5 tiles in a 1×10 grid, imagine adding an extra blank square to either the start or end of the grid or between two of the counters.
There are 6 places this tile could be inserted leading to 6 arrangements of 5 tiles in a 1×10 grid.
For 5 tiles in a 2×10 grid, you can first pick the columns the tiles go in (as a tile being in a column means nothing can be placed the columns either side, the number of ways to pick
columns is the same and the number of wats to arrange 5 tokens in a 1×10 grid). For each of these column choices, there are two locations for each tile (top or bottom).
This leads to a total number of arrangements of 6×25=192.
Tags: numbers, combinatorics
5 December
Carol rolled a large handful of six-sided dice. The total of all the numbers Carol got was 521. After some calculating, Carol worked out that the probability that of her total being 521
was the same as the probability that her total being 200. How many dice did Carol roll?
Show answer
Hide answer
The totals that are equally likely add up to 7 times the number of dice.
This can be seen by using the fact that the opposite sides of a dice add up to 7: for each way of making a given total with \(n\) dice, there is a way of making \(7n\) minus that total
by looking at the dice from below. Therefore \(T\) and \(7n-T\) are equally likely. (This also holds true (but is harder to explain) if you rearrange the faces of the dice so that the opposite
faces no longer add to 7.)
Therefore today's number is \((521+200)/7\), which is 103.
4 December
Today's number is a three digit number which is equal to the sum of the cubes of its digits. One less than today's number also has this property.
Show answer
Hide answer
If the final digit of the number is 0, then some carrying takes place when 1 is subtracted. Otherwise, no carrying happens.
If no carrying happens, call the three digits of today's number \(A\), \(B\), and \(C\). We know that \(A^3+B^3+C^3\) is one more than \(A^3 + B^3 + (C-1)^3\).
This implies that \(C^3=(C-1)^3+1\), which is only possible if \(C\) is 1.
Therefore either the final digit of today's number is 0 or the final digt of one less that today's number is 0. In both cases, we need to find a number
with the desired property whose final digit is 0: we are looking for digit \(A\) and \(B\) such that \(A^3+B^3\) is a multiple of 10.
Looking at all the cube numbers, there are a few combinations that add up to multiple of 10:
$$0^3+0^3=0$$
$$1^3+9^3=730$$
$$2^3+8^3=520$$
$$3^3+7^3=370$$
$$4^3+6^3=280$$
$$5^3+5^3=250$$
The only one of these that has the required property is 370. By checking 369 and finding it doesn't have the property, we see that the two numbers must be
370 and 371.
3 December
Put the digits 1 to 9 (using each digit exactly once) in the boxes so that the sums are correct. The sums should be read left to right and top to bottom ignoring the usual order of operations. For example, 4+3×2 is 14, not 10.
Today's number is the largest number you can make with the digits in the red boxes.
| ÷ | - | = 3 | |||
| + | + | ÷ | |||
| ÷ | × | = 1 | |||
| × | - | + | |||
| - | × | = 20 | |||
| = 91 | = 6 | = 8 |
Show answer
Hide answer
| 9 | ÷ | 1 | - | 6 | = 3 |
| + | + | ÷ | |||
| 4 | ÷ | 8 | × | 2 | = 1 |
| × | - | + | |||
| 7 | - | 3 | × | 5 | = 20 |
| = 91 | = 6 | = 8 |
The largest number you can make with the digits in the red boxes is 321.
2 December
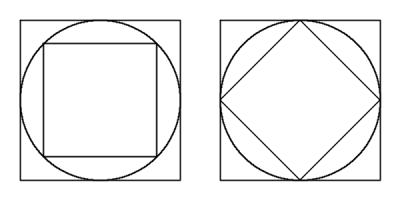
Carol draws a square with area 62. She then draws the smallest possible circle that this square is contained inside.
Next, she draws the smallest possible square that her circle is contained inside. What is the area of her second square?
Show answer
Hide answer
By drawing an appropriate diagram, it can be seen that the small square has half the area of the large square.
Therefore the area of the large square is 124.
1 December
It is possible to write 325 different numbers using the digits 1, 2, 3, 4, and 5 at most once each (and using no other digits).
How many of these numbers are odd?
Show answer
Hide answer
There are 3 one-digit numbers using these digits (1, 3 and 5).
To make two-digit odd numbers, there are 3 choices for the units digit, and 4 remaining choices for the tens digit.
To make three-digit odd numbers, there are 3 choices for the units digit, 4 remaining choices for the tens digit, and 3 remaining choices for the hundreds digit.
To make four-digit odd numbers, there are 3 choices for the units digit, 4 remaining choices for the tens digit, 3 remaining choices for the hundreds digit, and 2 remaining choices for the thousands digit.
To make five-digit odd numbers, there are 3 choices for the units digit, 4 remaining choices for the tens digit, 3 remaining choices for the hundreds digit, 2 remaining choices for the thousands digit and 1 remaining choice for the ten-thousands digit.
In total, this gives 3 + 3×4× + 3×4×3 + 3×4×3×2 + 3×4×3×2×1 = 195 odd numbers.
End of page. Show older puzzles.
Archive
Show me a random puzzle Most recent collections
Advent calendar 2024
Advent calendar 2023
Advent calendar 2022
Advent calendar 2021
List of all puzzles
Tags
tangents shape pentagons remainders routes quadratics sequences perimeter palindromes averages dodecagons dice rectangles coins 2d shapes consecutive numbers planes cryptic clues division lines integers square grids cube numbers even numbers axes complex numbers products decahedra elections doubling advent gerrymandering trigonometry books means regular shapes taxicab geometry mean perfect numbers dates surds sport binary irreducible numbers volume people maths arrows quadrilaterals matrices ave spheres polygons partitions functions odd numbers games digits addition logic balancing tournaments triangles proportion triangle numbers consecutive integers sum to infinity integration cryptic crossnumbers expansions indices calculus cubics speed wordplay unit fractions numbers grids hexagons dominos shapes neighbours angles determinants sums symmetry christmas square roots factorials 3d shapes range coordinates chess fractions star numbers number albgebra bases probability scales time geometric mean digital clocks cards digital products folding tube maps median combinatorics geometry powers multiplication square numbers pascal's triangle numbers parabolas ellipses area the only crossnumber chalkdust crossnumber medians geometric means percentages multiples squares crosswords algebra rugby menace clocks money polynomials floors circles factors prime numbers differentiation probabilty sets grids colouring crossnumbers graphs tiling chocolateArchive
Show me a random puzzle▼ show ▼ ▲ hide ▲
Most recent collections
Advent calendar 2024
Advent calendar 2023
Advent calendar 2022
Advent calendar 2021
List of all puzzles
Tags
partitions perfect numbers differentiation taxicab geometry balancing circles graphs games sets dodecagons symmetry geometric means range cube numbers regular shapes perimeter squares chocolate sequences pascal's triangle cryptic clues geometric mean lines determinants routes advent factorials numbers grids medians fractions gerrymandering odd numbers shape multiples rugby cubics consecutive integers books addition calculus dates factors volume sum to infinity christmas multiplication probability polygons digital clocks tangents coins arrows binary remainders chalkdust crossnumber unit fractions quadrilaterals scales even numbers menace cryptic crossnumbers tiling palindromes spheres consecutive numbers 2d shapes division colouring percentages parabolas people maths ellipses wordplay money quadratics mean cards averages doubling probabilty square grids products crosswords decahedra dice time logic speed expansions angles axes functions geometry digits integration complex numbers trigonometry shapes surds combinatorics albgebra indices number tournaments digital products star numbers neighbours clocks chess hexagons sums square numbers coordinates prime numbers rectangles sport planes crossnumbers triangles the only crossnumber ave elections pentagons polynomials area floors bases algebra irreducible numbers matrices grids numbers proportion means square roots integers 3d shapes dominos median powers triangle numbers folding tube maps© Matthew Scroggs 2012–2025