Puzzles
Bézier curve
A Bézier curve is created as follows:
![](javascript:showlimage("bezier.gif"))
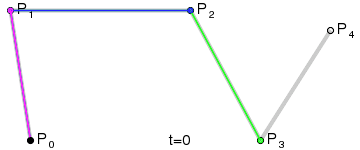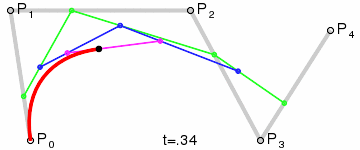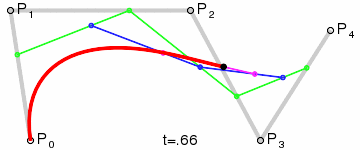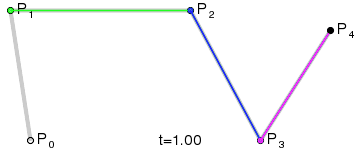
1) A set of points \(P_0\), ..., \(P_n\) are chosen (in the example \(n=4\)).
2) A set of points \(Q_0\), ..., \(Q_{n-1}\) are defined by \(Q_i=t P_{i+1}+(1-t) P_i\) (shown in green).
3) A set of points \(R_0\), ..., \(R_{n-2}\) are defined by \(R_i=t Q_{i+1}+(1-t) Q_i\) (shown in blue).
.
.
.
\(n\)) After repeating the process \(n\) times, there will be one point. The Bézier curve is the path traced by this point at \(t\) varies between 0 and 1.
What is the Cartesian equation of the curve formed when:
$$P_0=\left(0,1\right)$$
$$P_1=\left(0,0\right)$$
$$P_2=\left(1,0\right)$$If you enjoyed this puzzle, check out Sunday Afternoon Maths XX,
puzzles about coordinates, or a random puzzle.
puzzles about coordinates, or a random puzzle.